Listen to this article

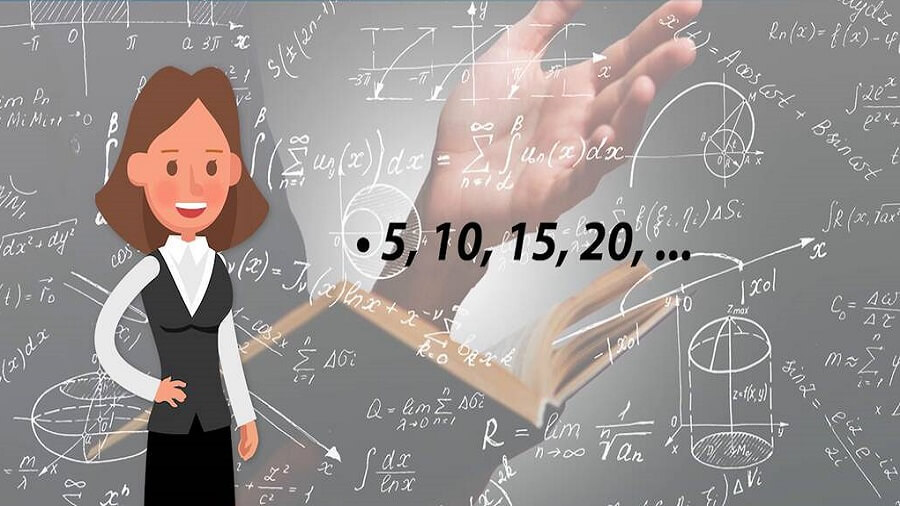
Introduction

An arithmetic sequence is a mathematical term that refers to a set of numbers in which each number is obtained from the previous one by adding or subtracting a fixed amount. Such series are called arithmetic because they involve whole-number addition, subtraction, multiplication, or division with no remainder. The word ‘arithmetic’ is derived from the Greek meaning ‘the things added’. The term arithmetic sequence is used to refer to both the set of numbers and the relationship between them.

What is an Arithmetic Sequence?

In mathematics, an arithmetic sequence is a sequence of numbers in which each term is obtained by adding a fixed amount to the previous term. The first term is called the first term, the common difference is called the common difference and each succeeding term after that has its name.

For example: 1 2 4 8 16 32 64 128 256 512 1024 2048 4096 8192 16384 32768 65536 131072 262144 524288 1048576 2097152 4194304 8388608 16777216 33554432 67108864 13421728

Arithmetic Sequence Example

An arithmetic sequence is a sequence of numbers in which each term is the sum of all preceding terms. For example, 1, 4, 9, and 16 are arithmetic sequences because they add up to 27.

An example of an arithmetic sequence with negative terms is: -1,-2,-3,-4, etc., where -1 means “minus one” and every subsequent number represents an increase in magnitude by one unit. So if you want to find out how many negative terms there are in this series, just take away its first term (i.e., subtract 1). This will tell you how many negative terms there are in total between 0 and 1 inclusive; that would be 3!

Arithmetic Sequence Formula

The arithmetic sequence formula is a way to calculate the sum of an arithmetic series. It is also known as the recursive formula and can be used to find the sum of any finite number of terms in an arithmetic sequence, including:

• The first term (1), which is not included in the sum
• The last term without its multiplicand(s)

Nth Term of Arithmetic Sequence

The nth term of an arithmetic sequence is equal to the sum of the first n terms. Mathematically, it can be written as:

$$\sum_{n=1}^{n}x_n$$

or more simply as: $$\sum_{n=1}^{n}\frac{x_1}{1+x_1}$$

The formula can be rewritten as:$$\sum_{n=1}^{n}\frac{x_1}{1+x_1}=\frac{x_1}{(1+x_1)}$$ or even more simply as:$$\frac{x_2}{(1+x_2)}=\frac{x_1}{(1+x_1)} The formula can be rewritten as:$$\sum_{n=1}^{n}\frac{x_1}{1+x_1}=\frac{x_1}{(1+x_1)}$$ or even more simply as:$$\frac{x_2}{(1+x_2)}=\frac{x_1}{(1+x_1)} 

Arithmetic Series

For example, 2+3+5=12 and 4+6+7=21. If you add these two numbers together, you get 21 as a result. Therefore, the sum of the first n terms of an arithmetic sequence can be written as:

i)The common difference between arithmetic sequences can be either positive or negative or zero. ii)The common difference between arithmetic sequences is the positive difference between any two consecutive terms in that sequence. For example, in the first arithmetic series above, the common difference is 3.

ii)The sum of the first n terms of an arithmetic sequence is called as the arithmetic series.

I ii)The common difference between arithmetic sequences can be either positive or negative or zero. The sum of the first n terms of an arithmetic sequence is called as the arithmetic series. The sum of the first n terms of an arithmetic sequence is called as the arithmetic series. 

Important Notes on Arithmetic Sequence

The arithmetic sequence is a sequence in which the difference between consecutive terms is constant.

The difference between consecutive terms is called a common difference and it is always positive or zero. The first term of an arithmetic sequence can be written as a + b where a and b are real numbers, and then each succeeding term will be obtained by adding another factor to the previous term:

(a + b)^n = (a + b)^n-1 + … + (a + b)^0 = a^(n-1).

Conclusion

We hope you enjoyed learning more about arithmetic sequences and how they can be used in your daily life. In case you’re interested in seeing more examples of arithmetic sequences, please check out our other posts on the topic from this site. We also have a whole section of our site dedicated to helping you learn more about arithmetic sequences and how they are used in real life. We hope this has been helpful!